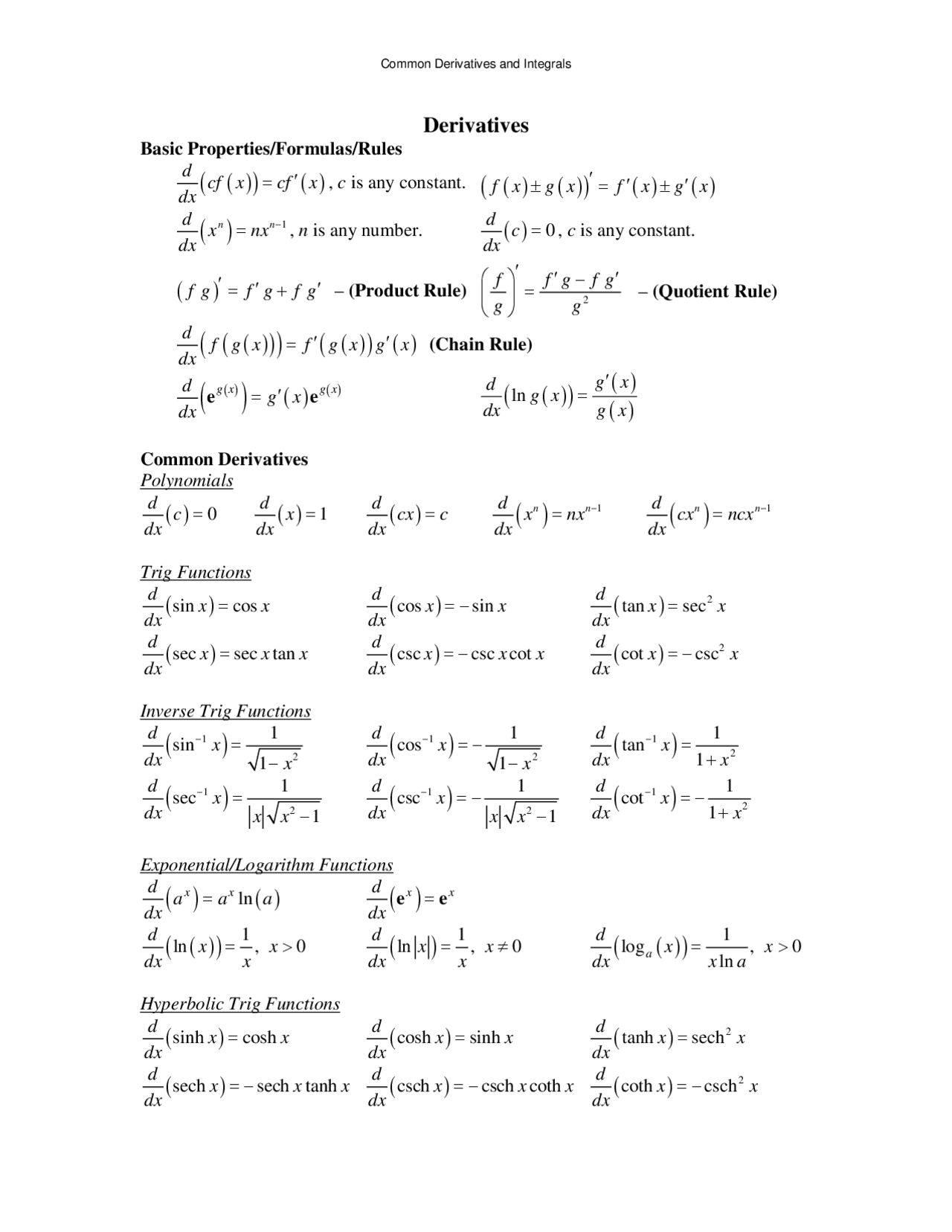
Is the world of calculus a maze of complex symbols and bewildering rules? Understanding the fundamental principles of derivatives can unlock a deeper comprehension of how things change, providing a powerful lens through which to view the world around us.
At its core, calculus, and specifically the concept of derivatives, provides a systematic way to examine the rate of change of a function. It allows us to dissect curves, understand the velocity of moving objects, and even optimize processes in various fields, from economics to engineering. While the initial encounter may seem daunting, mastering the derivative opens doors to a fascinating world of problem-solving and understanding.
This guide serves as a cheat sheet, a companion for navigating the world of derivatives, covering the essential rules and formulas necessary for success. It's your quick reference for understanding and applying the core principles, simplifying complex problems. The goal here is to make the seemingly complex approachable, demystifying the notation and concepts surrounding the derivative. Derivatives represent a fundamental concept in calculus. This document delves into the core definition of derivatives and provides insights into their notations, empowering you to engage with calculus more effectively. We will delve into the application of differentiation to trigonometric, inverse trigonometric, exponential, and logarithmic functions, crucial for understanding their behavior and applications across various fields.
- Melinda Clarke Bio Roles In The Oc Csi More Info News
- Unseen Dahmer Inside The Crimes Crime Scene Photos
Let's start with the definition of the derivative. For a function, y = f(x) , the derivative, often denoted as f'(x) or dy/dx, is defined as the limit of the difference quotient: f'(x) = lim (h0) [f(x + h) - f(x)] / h . This formula captures the instantaneous rate of change of the function f(x) at a given point x. Another way to put it is: the rate of change of f(x) with respect to x.
Here's a summary of alternative notation for the derivative, all being completely equivalent and useful for different contexts:
f'(x)
- Roberto Esquivel Cabrera Worlds Longest Penis Is It A Curse
- Steven Greener Meet Tamron Halls Husband Music Exec
y'
dy/dx
d/dx [f(x)]
The power rule is fundamental: For a function of the form f(x) = x^n, the derivative is f'(x) = nx^(n-1). This rule is your primary tool for differentiating polynomial functions. Another rule is the product rule, used when differentiating the product of two functions. The product rule tells you how to find the derivative of the product of two functions. If you're trying to find the derivative of f(x) = u(x)v(x), then the derivative f'(x) = u'(x)v(x) + u(x)v'(x), the derivative of the first function times the second function plus the first function times the derivative of the second. The quotient rule, a derivative rule that addresses a quotient of two functions. It is applicable when differentiating a function that is the ratio of two functions. So, for a function of the form f(x) = u(x) / v(x), then f'(x) = [u'(x)v(x) - u(x)v'(x)] / [v(x)]^2. The chain rule is used to differentiate a composite functiona function within a function. Given a function, f(x) = g(h(x)), the chain rule is f'(x) = g'(h(x)) h'(x) .
Here's a quick reference for the derivative of some common functions that can be found in most calculus textbooks.
We now go to the derivatives for the Trigonometric functions:
If f(x) = sin(x) , then f'(x) = cos(x)
If f(x) = cos(x) , then f'(x) = -sin(x)
If f(x) = tan(x) , then f'(x) = sec^2(x)
If f(x) = csc(x) , then f'(x) = -csc(x)cot(x)
If f(x) = sec(x) , then f'(x) = sec(x)tan(x)
If f(x) = cot(x) , then f'(x) = -csc^2(x)
Inverse Trigonometric Functions:
If f(x) = sin^-1(x) , then f'(x) = 1 / sqrt(1 - x^2)
If f(x) = cos^-1(x) , then f'(x) = -1 / sqrt(1 - x^2)
If f(x) = tan^-1(x) , then f'(x) = 1 / (1 + x^2)
If f(x) = csc^-1(x) , then f'(x) = -1 / (|x| sqrt(x^2 - 1))
If f(x) = sec^-1(x), then f'(x) = 1 / (|x|sqrt(x^2 - 1))
If f(x) = cot^-1(x) , then f'(x) = -1 / (1 + x^2)
Exponentials and Logarithms:
If f(x) = e^x , then f'(x) = e^x
If f(x) = a^x , then f'(x) = a^x ln(a)
If f(x) = ln(x), then f'(x) = 1/x
If f(x) = log_a(x), then f'(x) = 1 / (x ln(a))
Derivatives are also vital for understanding inverse trigonometric, hyperbolic, and inverse hyperbolic functions, providing crucial tools for solving complex problems in calculus and its applications.
Here's a table summarizing the derivatives and integrals for a select set of common functions. This is by no means an exhaustive list, but is a great starting point. Please note, the integral has a constant of integration 'C' because the derivative of a constant is zero:
| Function, f(x) | Derivative, f'(x) | Integral, f(x) dx |
|---|---|---|
| x^n | nx^(n-1) | (x^(n+1))/(n+1) + C |
| sin(x) | cos(x) | -cos(x) + C |
| cos(x) | -sin(x) | sin(x) + C |
| tan(x) | sec^2(x) | ln|sec(x)| + C |
| e^x | e^x | e^x + C |
| ln(x) | 1/x | xln(x) - x + C |
| a^x | a^xln(a) | a^x/ln(a) + C |
| arcsin(x) | 1/sqrt(1-x^2) | x arcsin(x) + sqrt(1-x^2) + C |
| arccos(x) | -1/sqrt(1-x^2) | xarccos(x) - sqrt(1-x^2) + C |
| arctan(x) | 1/(1+x^2) | x arctan(x) - 1/2 ln(1+x^2) + C |
| sec(x) | sec(x) tan(x) | ln|sec(x)+tan(x)| + C |
| csc(x) | -csc(x)cot(x) | ln|csc(x)-cot(x)| + C |
| cot(x) | -csc^2(x) | ln|sin(x)| + C |
| sinh(x) | cosh(x) | cosh(x) + C |
| cosh(x) | sinh(x) | sinh(x) + C |
| tanh(x) | sech^2(x) | ln(cosh(x)) + C |
| sech(x) | -sech(x) tanh(x) | 2arctan(e^x) - x + C |
| csch(x) | -csch(x)*coth(x) | ln|tanh(x/2)| + C |
| coth(x) | -csch^2(x) | ln|sinh(x)| + C |
- Gracie Mae Thompson Scam What You Need To Know Stay Safe
- Milan Mirabella Ethical Concerns Search Results Discover Guide